We make the approximation that exactly two light beams of equal intensity reach the observer. One beam is reflected from the first glass-air interface, and the other beam is reflected from the second glass air interface. We also ignore any reflections except at these two interfaces. The index of refraction of glass is greater than that of air, therefore, the beam that is reflected from the first glass-air interface will not undergo a phase shift but the beam that is reflected from the second glass-air interface will undergo a phase shift. Therefore, the phase difference between the two beams is
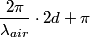 where 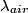 is the wavelength of light in air and is the wavelength of light in air and 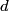 is the thickness of the film of air. We are given that is the thickness of the film of air. We are given that 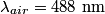 . In order for constructive interference to occur, the phase difference must equal . In order for constructive interference to occur, the phase difference must equal  where where 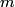 is an integer. Therefore, is an integer. Therefore,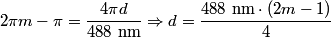 We can evaluate 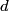 for for 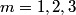 to find that to find that 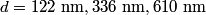 . Therefore, answer (E) is correct. . Therefore, answer (E) is correct. |
Please send questions or comments to X@gmail.com where X = physgre.